11.17.2018: One Lesson of Math - Interpreting the Discriminant

Today's soundtrack is Shawn McDonald: Simply Nothing. "Take My Hand" is by far my favourite track on the album; it brings me back many years to a time of seemingly endless possibilities.

We can interpret the discriminant of a quadratic equation to determine the number of roots that a quadratic equation has - without needing to solve the equation!

When we substitute a quadratic equation in the form of ax² + bx + c = 0 into the quadratic formula, the section inside the radical, b² - 4ac, tells us how many real roots the equation has. We use the delta symbol, Δ, to represent b² - 4ac, and we can determine the number of roots in a quadratic equation based on Δ by using the following information: ​​

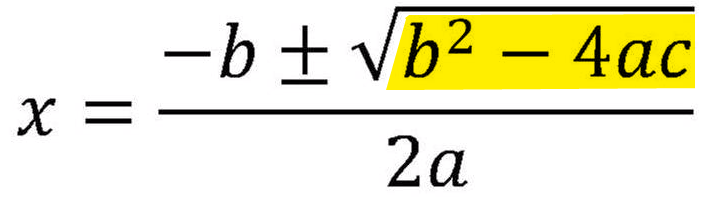
- If Δ > 0, the equation has two real roots

- If Δ = 0, the equation has one real root

- If Δ < 0, the equation has no real roots

- if Δ = a perfect square, it is rational, and we can solve by factoring

- if Δ ≠ a perfect square, it is irrational, and it cannot be solved by factoring

#ShawnMcDonald